In a nutshell. Well, theories of any subject benefit true from a rock or two hard spots to build a legacy on yes ? In music, our aural bedrock is as perfect as nature can provide, all part of the 'grand plan' for the perfect harmony potentials for all sentient beings. For that is what the octave interval sounds for us; the perfect merge of two pitches into one beautiful and unbreakable musical sound. Upon this perfection musical sound we have and continue build and build and build and ... :)

Why the number Eight? We as theorists use this number 'eight' as it caps the octave pitch atop the seven pitches of the the diatonic relative major / minor group, by far and away, our most beloved loop of pitches for creating the wide range of Americana musics. Examine the following chart that matches letter pitches in C major and corresponding scale degree numbers as we locate the 'octave cap' of Eight. Example 1.

Easy enough huh? By now you're probably able to do this in your sleep. Rote learned? 12 keys? Thus the ways and means, methods and madness of the music theorist.

Theory names; octave, Eight, Ionian mode. We've reached the close of what we term are simple intervals, those that live within an octave span. So in most of the theory, all things One are also all things Eight, we're just up or down an octave as the case might be. Examine the octave interval from our root pitch C. Do learn to sing the octave really strong and confidently from any pitch :) Example 1a.

Octave doubling in chords. The C major chord tabbed above is sometimes known as the 'solid C.' In all probability named due to its ability to take a physical pounding with our strumming hand, for we get to use all six of the strings but only sound three different pitches. Imagine that :) A lot of the basic open chords feature this doubling, very common really. Examine the pitches from lowest to highest. Example 1b.

Each of the three pitches in our chord are doubled in octaves. Its bass note G makes this chord a 'second inversion' chord. Many of our most common chord shapes, especially open chords for the folk styles, use this octave doubling to firm things up. Many of our barre chord shapes too.

In our personal harmony evolutions with chords, when we start to use chord voicings without any doubling of their pitches, then we're probably moving beyond triads and adding in the color tones, thus moving in a blues / rock / country / pop and jazz directions.

Artists whose music moves into writing for larger groups, orchestras, wind ensembles, scoring for films and theatre etc., will surely run into this octave doubling at some point. Our loud and soft dynamic markings often go just so far in getting our musical points across. Getting lots of voices doubled on the root pitch can not only settle things down in a hurry but provide a warmth and restive depth of peace we've come to love in our musics.

So why so important? Well as we can hear from the sounds, octave doubling can absolutely solidify the sound of any aspects of our music. And in our Americana music, the 'heavier' the sound, especially in the rock styles, the more doubling we'll probably encounter. Conversely, as we lessen or avoid doubling pitches, we can lighten the texture and sound of the music. Lighter can go faster, so heading towards jazz.

Octave interval melodies. There are quite a number of melodies that contain the octave interval. We often muse about how melodies with an octave interval somewhere in their line always seem to have some sort of extra coolness. While these days mostly a pop and jazz thing, here's the first phrase of an Americana classic that opens with the octave interval. Example 1c.

Great line huh? Ya gotta know it by heart right? Find it by ear on your ax through a couple of key centers.

Melodic doubling. A very cool and potentially very exciting thing we can do is simply play our melody lines with an octave doubling. Guitarists often do this. And really any combination of instruments can do this too. This next idea reaches back to the core of it all Americana. Example 2.

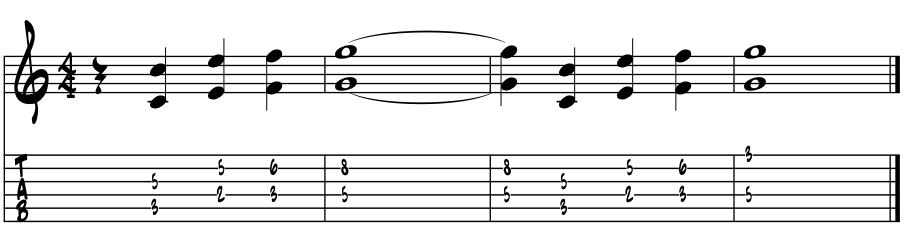Well, not an overly dramatic recreation of the effect admitted. But the basic idea of octave doubling is brought out in the example and a fingering solution for the pitches. Play the rest by ear, it's a great line to quote. And there's a chart and sound file of the whole tune if needed.

We can apply this doubling technique to all sorts of licks; scales arpeggios, sequences etc. While mostly a jazz guitar thing, we do hear it once in a while with the rockers and blues cats. Duane Allman used it to great effect and I think he used a pick to create the octave sound, fanning the strings. A lot of cats use the thumb to sound the octaves which with flat wound strings, makes their sound very warm so leaning jazz.

Jazz great Wes Montgomery made this octave style of melody playing a solid part of his signature sound. In the next generation, jazz and pop great George Benson, who followed suit, not only doubled his melodies in octaves but at times slipped a fifth, or other intervals, in between. Benson moves a step further in scat singing along with his lines. With or without octave doubling, Mr. Benson's vocal doubling creates some very exciting music. Many of today's monsters include this octave doubling in their approach to bringing their melodic inventions and interpretations to new life.

Review. The octave interval provides the purity of sound upon which our entire theoretical architecture is based upon. Nearly everything we find related to One carries over to Eight, which is simply up an octave. Eight becomes the new One if we continue stepwise.

That we guitarists often use octave doubling to enhance the strength of our sound. Arrangers of all musics count on the octave doubling to really bring a solidity to their ideas when needed. And the flip side, that reducing the amount of octave doubling in our voicings lightens the texture of the sound and opens up the upper arpeggio pitches and chord related color tones.

Pop quiz. Why are 'perfect' intervals termed perfect?

__________________________________________.

Why perfect? Simply because they sound the best. Of the dozens of different intervals we have, the octave interval is tops, period. For in the days of their devising, something 'perfect' was the word to describe when no equal of purity and beauty could be found to compare. Like groovy, cool and now awesome today? Yep, pretty much. That of all of the many many interval combinations we can conjure up and aurally sound on real, consistently tuneable instruments then and today, the 'perfect' intervals sound and blend the best together.

These perfect intervals are the base pitches of the naturally occurring overtone series and center developing a major / minor key center, the perfect intervals become the root pitches of all things One / Four / Five; scale degrees, arpeggios, triads and chords with color tones. That there's a numerical math process that supports such 'perfection' of musical tone is no surprise really, as Mother Nature already knows all the natural, organic magics, we then come along and 'discover' the math and magics anew :)